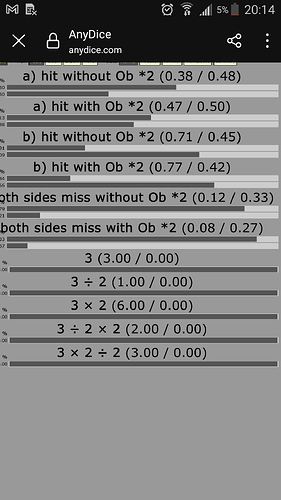
Here’s the orthodox method:
Ob penalties apply first, directly to your unmodified die pool; They’re not affected by Ob×2. Do not add your Ob penalties to the other side’s successes.
Beginner’s Luck (or other Ob×2 penalty) halves your successes in a versus test. Discard any fractional successes. This is the method prescribed in the Codex.
Do not double the opponent’s successes. Do not apply Beginner’s Luck Ob×2 when the obstacle does not require a skill, e.g. your testing stat vs stat—including BL vs BL. (A good way of looking at this is that you can test a stat instead of a skill and lacking the required skill for the obstacle imposes Ob×2.)
Margin of success is the number of successes you have in excess of passing or winning the test. It’s the number of successes you could lose without changing the basic result.
Therefore:
Lucky rolls Agility 3 +1 Ob versus Skilly’s Sword 2. The +Ob knocks Lucky down to 2, then Ob×2 cuts that in half to 1 succes. Swordy’s 2 successes stay just like that. Swordy wins, 0 margin (losing any successes would result in a tie).
Lucky calculates the Ob for advancement as Swordy’s 2 successes plus his +1 Ob, for 3, which is as you say routine and counts toward opening Sword.
BQ 1: Neither side suffers Ob×2.
BQ 2: Neither side suffers Ob×2.
In both Bonus Question cases, the characters testing Beginner’s Luck count routine tests toward opening the skill.
Regarding opponent’s Ob penalties and advancement, they reduce the effective Ob just like they reduce your opponent’s pool of successes. 4s +1 Ob = 3s = Ob 3 for advancement.
This doesn’t make any sense. If you didn’t apply the Ob×2 (to either side) it would be 3 vs 2, the 3 would still win. In fact, if you apply Ob×2 to both sides, the basic result will never change. What does change is the margins (if you double a difference of 1, it becomes a difference of 2).
Unless you calculate versus tests as simultaneous standard tests, which is not recommended anywhere in the books. (I’ve tried it, too, and ultimately it lacks utility to make up for the complexity it introduces.) And Bloody Versus is two simultaneous versus tests as a specific subsystem; It should not be taken as the standard for versus tests.
To summarize, the simple, “standard” approach:
- Roll ze dice
- Subtract Ob penalties
- Assess Double Ob when the obstacle requires a skill, not just because you’re activating Beginner’s Luck
- Halve for Double Ob, discard half-successes
- Compare, the side with more wins (or there’s a tie)
- Margin of Success: how many successes the winner could lose and still win
-:-
Now, this is not the only way to do it. In fact, you will need to either interpret the rules to match your method or employ a different method for contradictory cases—Margin in the Duel of Wits rules refers to all of your effective successes, including the one you needed to win, while the Fight rules count only the extras.
The Fight rules imply that you literally double the opponent’s successes then add your Ob penalty to that total. Which means if you, unskilled, are standing and drooling and superficially injured and get punched by a marine with B3 Brawling, the worst he can do is a Mark hit—but if you tried to block (inadvisable) and rolled 1, he could roll 3, double to 6, add 1 for your supe, and hit you with a Superb that he can aim, too. (Unless you count that first success outside the margin, then he can’t aim. See how fiddly this gets?)
So, if you try to do anything, instead of standing there like an inanimate object, you are likely to get hit harder. Does that make sense? It certainly seems to discourage unskilled folks from trying anything. That’s the case against literally doubling the other guy’s successes.
My preferred method for handling unskilled vs. skilled is a little different from the orthodox.
Here’s a table (top: skilled successes, left: unskilled successes):
| A |
1 |
2 |
3 |
4 |
5 |
| 1 |
S+0 |
S+1 |
S+2 |
S+3 |
S+4 |
| 2 |
Tie |
S+0 |
S+1 |
S+2 |
S+3 |
| 3 |
U+0 |
S+0 |
S+1 |
S+2 |
S+3 |
| 4 |
U+1 |
Tie |
S+0 |
S+1 |
S+2 |
| 5 |
U+2 |
U+0 |
S+0 |
S+1 |
S+2 |
The math I use to get there is whatever is expedient but the principle is: The mark is what you need to tie with the other guy. If you have more than that, you win. For every extra success, it’s +1 MoS. If you lose and your MoF matters, it’s calculated from your perspective: One less than you needed to meet the mark is MoF 1, and so on.
Why do I do this? I did a lot of math and got into an argument with Quincy about it. It’s so that skilled characters matched with unskilled characters with twice the dice have the same odds of success and their margins are counted the same. The unskilled characters’ luck is chaotic, just as likely to accidentally blow away the obstacles as they are to fail abysmally. The skilled characters can’t achieve the same heights by sheer beginner’s luck anymore, but they are consistently better in the margins than the unskilled: Always failing by a little bit, or oversucceeding by a little bit.
Throwing out half successes changes all this. Unskilled characters are simply more likely to fail, to do so catastrophically, and when they succeed, it is by a slim margin. I don’t like the way that feels, so I prefer my way.
Here’s the table for the orthodox way, for comparison (top: skilled successes, left: unskilled successes):
|
1 |
2 |
3 |
4 |
5 |
| 1 |
S+0 |
S+1 |
S+2 |
S+3 |
S+4 |
| 2 |
Tie |
S+0 |
S+1 |
S+2 |
S+3 |
| 3 |
Tie |
S+0 |
S+1 |
S+2 |
S+3 |
| 4 |
U+0 |
Tie |
S+0 |
S+1 |
S+2 |
| 5 |
U+0 |
Tie |
S+0 |
S+1 |
S+2 |
Skilly’s results have stayed the same, but now Unskilled can’t hope to do better than tie or make a bare success. I think the message is clear: Don’t do shit unskilled.
Or can I hope for a bundle sale in the store any time? 
Anyway, thanks a lot for this brilliant game and the cool forum! :*
Thanks!